Calorimetry Formulas

1. Specific heat capacity

Is defined as the amount of heat required to raise the temp, of unit mass of a body by 1┬░C
s = \(\frac{\mathrm{Q}}{\mathrm{m} \Delta \theta}\)
unit ΓåÆ J/kg – ┬░C (S. I. system)
→ cal/gm-°C

2. Heat capacity (C)

C = ms = \(\frac{\mathrm{Q}}{\Delta \theta}\)

3. Water equivalent

When the heat capacity of a body is expressed in terms of mass of water, it is called water equivalent of the body. It is the mass of water which when given same heat as to the body, changes the temperature of water through the same range as that of the body.
W = ms

4. Latent heat

(a) The heat required to change the unit mass of a solid to its liquid state at its melting point is called Latent Heat of Fusion. For changing from ice to water L_f = 80 cal/gm.

(b) The heat required to change the unit mass of the liquid to its vapour state at its boiling points is called Latent Heat of Vapourisation. For changing from water to steam L_v = 540 cal/gm.

(c) Q = mL

Note:-
1. For changing from solid (ice) to gas (steam), following transformation takes place. Suppose you have an ice at 2┬░C and you want to convert it to steam at 100┬░C than,


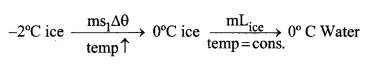
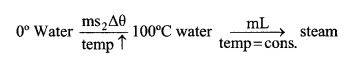
It can also be shown graphically

5. Principle of calorimetry

(i) When two substances of different temperatures are mixed.
Heat released by I substance ΓÑñ Heat absorbed by II substance
m₁s₁ (T₁ – T) = m₂s₂ (T – T₁)
Final temp T = \(\frac{m_{1} s_{1} T_{1}+m_{2} s_{2} T_{2}}{m_{1} s_{1}+m_{2} s_{2}}\)

6. Solution technique to find specific heat of a solid

Consider a calorimeter whose water equivalent is W. Assume that it contains mass m, of water at temperature ╬╕₁. A hot solid at temperature ╬╕₂ having mass m₂ is placed in the calorimeter. The mixture acquires a final temperature ╬╕.
If s is specific heat of water, then
Heat gained by water and calorimeter = (m₁ + W)s ├ù (╬╕ – ╬╕₁)
If s’ is specific heat of solid, then
Heat lost by solid = m₂ ├ù s’ ├ù (╬╕₂ – ╬╕)
by principle of calorimetry,
m₂s’ (╬╕₂ – ╬╕) = (m₁ + W)s (╬╕ – ╬╕₁)
s’ = \(\frac{\left(m_{1}+W\right) s\left(\theta-\theta_{1}\right)}{m_{2}\left(\theta_{2}-\theta\right)}\)

7. To find the latent heat of vapourisation

Consider a calorimeter of water equivalent W. Assume it to contain water at temperature ╬╕₁ having mass m₁. Suppose the steam at temperature ╬╕₂ of mass m₂ is passed through water so that temperature of the mixture becomes ╬╕.
If s is the specific heat of water, then.
Heat gained by water and calorimeter = (m₁ + W) ├ù s ├ù (╬╕ – ╬╕₁)
If L is the latent heat of vapourisation, then
Heat lost by steam in changing to water = m₂L
Heat lost by water = m₂ ├ù s ├ù (╬╕₂ – ╬╕)
by principle of calorimetry
Heat lost = Heat gained
m₂L + m₂s (╬╕₂ – ╬╕) = (m₁ + W) s (╬╕ – ╬╕₁)
m₂L = (m₁ + W) s (╬╕ – ╬╕₁) – m₂s (╬╕₂ – ╬╕)
L = \(\frac{\left(m_{1}+W\right) s\left(\theta-\theta_{1}\right)}{m_{2}}\) – s(╬╕₂ – ╬╕)

8. Mechanical equivalent of heat (J)

W = JH Unit of J = Joule/cal.
Γåæ Γåæ J = 4.2 J/cal.
In joule In calorie

9. Conversion of temp. scale

\(\frac{\mathrm{T}_{\mathrm{C}}-0}{100}=\frac{\mathrm{T}_{\mathrm{F}}-32}{180}=\frac{\mathrm{T}_{\mathrm{R}}-0}{80}=\frac{\mathrm{T}_{\mathrm{K}}-273}{100}\)
⇒ \(\frac{T_{C}}{5}=\frac{T_{F}-32}{9}=\frac{T_{R}}{4}=\frac{T_{K}-273}{5}\)
\(\frac{\Delta \mathrm{T}_{\mathrm{C}}}{5}=\frac{\Delta \mathrm{T}_{\mathrm{F}}}{9}=\frac{\Delta \mathrm{T}_{\mathrm{R}}}{4}=\frac{\Delta \mathrm{T}_{\mathrm{K}}}{5}\)
Δ → change
T_C → Temp.in Celsius
T_F → Temp.in Farenheit
T_R → Temp.in Reaumer
T_K → Temp.in Kelvin

Understand the concepts of several physics concepts all under one roof at Physicscalc.Com and get a good hold of the concept.