Laws of Conservation Formulas

If \(\overrightarrow{\mathrm{F}}=\mathrm{F}_{\mathrm{x}} \hat{\mathrm{i}}+\hat{\mathrm{i}} \text { Fy } \hat{\mathrm{i}}+\mathrm{F}_{\mathrm{z}} \hat{\mathrm{k}}\)
& \(\overrightarrow{\mathrm{P}}=\mathrm{P}_{\mathrm{x}} \hat{\mathrm{i}}+\hat{\mathrm{i}} \text { Py } \hat{\mathrm{i}}+\mathrm{P}_{\mathrm{z}} \hat{\mathrm{k}}\)
F_x = \(\frac{\mathrm{dP}_{\mathrm{x}}}{\mathrm{dt}}\) if F_x = 0 , then P_x = constant
F_y = \(\frac{\mathrm{dP}_{\mathrm{y}}}{\mathrm{dt}}\) if F_y = 0, then P_y = constant
F_z = \(\frac{\mathrm{dP}_{\mathrm{z}}}{\mathrm{dt}}\) if F_z = 0, then P_z = constant

1. Important formula and features for direct elastic collision / head on elastic collision in id

(i) The velocity of first body after collision
v₁ = \(\left(\frac{m_{1}-m_{2}}{m_{1}+m_{2}}\right) u_{1}+\left(\frac{2 m_{2}}{m_{1}+m_{2}}\right)\)u₂

(ii) The velocity of second body after collision
v₂ = \(\left(\frac{2 m_{1}}{m_{1}+m_{2}}\right) u_{1}+\left(\frac{m_{2}-m_{1}}{m_{1}+m_{2}}\right)\)u₂

(iii) If the body with mass m₂ is initially at rest i.e. u₂ = 0
v₁ = \(\left(\frac{m_{1}-m_{2}}{m_{1}+m_{2}}\right)\)u₁
and v₂ = \(\left(\frac{2 m_{1}}{m_{1}+m_{2}}\right)\)u₁

(iv) When a particle of mass m₁ moving with velocity u₁, collides with another particle with m₂ at rest and if.
m₁ = m₂ m₁ >> m₂ m₁ << m₂
ΓçÆ v₁ = 0 ΓçÆ v₁ = u₁ ΓçÆ v₁ = -u₁
and v₂ = u₁ and v₂ = 2u₁ and v₂ = \(\frac{2 m_{1}}{m_{2}}\)u₁ Γëê 0

(v) When m₁ = m₂ = m but u₂ Γëá 0, then v₁ = u₂ and v₂ = u₁ i.e. the particles mutually exchange their velocities.

(vi) Exchange of energy is maximum when m₁ = m₂. This fact is utilized in atomic reactor in slowing down the neutrons. To slow down the neutrons, these are made to collide with nuclei of almost similar mass. For this hydrogen nuclei are most appropriate.

2. Head on inelastic collision

(a) In this case Total momentum before collision = Total momentum after collision
⇒ \(\mathbf{m}_{1} \overrightarrow{\mathbf{u}}_{1}+\mathbf{m}_{2} \overrightarrow{\mathbf{u}}_{2}=\mathbf{m}_{1} \overrightarrow{\mathbf{v}}_{1}+\mathbf{m}_{2} \overrightarrow{\mathbf{v}}_{2}\)
but K.E._b.c. Γëá K.E._a.c.
⇒ K.E._b.c. = K.E._a.c. + Q
where Q = heat energy, sound energy etc.
ΓçÆ \(\frac{1}{2}\) m₁u₁² + \(\frac{1}{2}\) m₂u₂² > \(\frac{1}{2}\) m₁v₁² + \(\frac{1}{2}\) m₂v₂²

(b) According to Newton’s law, for inelastic collision we have
\(\overrightarrow{\mathrm{v}}_{1}-\overrightarrow{\mathrm{v}}_{2}=-\mathrm{e}\left(\overrightarrow{\mathrm{u}}_{1}-\overrightarrow{\mathrm{u}}_{2}\right)\)

(c) In inelastic collision, velocity of first body after collision
v₁ = \(\left(\frac{m_{1}-e m_{2}}{m_{1}+m_{2}}\right) u_{1}+\frac{m_{2}(1+e)}{m_{1}+m_{2}} u_{2}\)
and v₂ = \(\frac{m_{1}(1+e)}{m_{1}+m_{2}} u_{1}+\frac{m_{2}-e m_{1}}{m_{1}+m_{2}} u_{2}\)

(d) Loss of energy in inelastic collision
╬öE_k = \(\frac{1}{2}\left(\frac{m_{1} m_{2}}{m_{1}+m_{2}}\right)\)(u₁ – u₂)²(1 – e²)

(e) Important Results:
(i) If e = 0 (perfect inelastic)
v₁ = v₂ = \(\frac{\mathrm{m}_{1} \mathrm{u}_{1}+\mathrm{m}_{2} \mathrm{u}_{2}}{\mathrm{m}_{1}+\mathrm{m}_{2}}\)
╬öE_k = \(\frac{1}{2} \frac{m_{1} m_{2}}{m_{1}+m_{2}}\)(u₁ – u₂)²

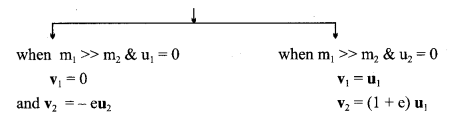
(ii) if m₁ >> m₂
then v₁ Γëâ u_1,
v₁ Γëâ (1 + e)u₁ – eu₂

3. Oblique collision

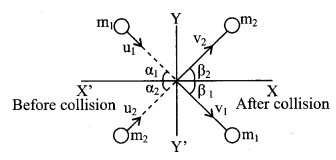
(a) Applying law of conservation of linear momentum along X axis
(P_x)_b.c. =(P_x)_a.c.
ΓçÆ m₁u₁ cos ╬▒₁ + m₂u₂ cos ╬▒₂= m₁v₁ cos ╬▓₁ + m₂v₂ cos ╬▓₁ and along y-axis
(P_y)_b.c. =(P_y)_a.c.
ΓçÆ m₁u₁ sin ╬▒₁ + m₂u₂ sin ╬▒₂= m₁v₁ sin ╬▓₁ + m₂v₂ sin ╬▓₁

(b) Applying law of conservation of K.E.
(K.E.)_b.c. = (K.E.)_a.c.
⇒ \(\frac{1}{2} \mathrm{m}_{1} \mathrm{u}_{1}^{2}+\frac{1}{2} \mathrm{m}_{2} \mathrm{u}_{2}^{2}=\frac{1}{2} \mathrm{m}_{1} \mathrm{v}_{1}^{2}+\frac{1}{2} \mathrm{m}_{2} \mathrm{v}_{2}^{2}\)

(c) If m₁ = m₂ and (╬▒₁ + ╬▒₂) = 90┬░, (╬▓₁ + ╬▓₂) = 90┬░ which means if two particles of same mass moving at right angles to each other collide elastically, after collision they move at right angles to each other.

(d) If a body A collides elastically with another body B of same mass at rest at a glancing angle, then after collision the two bodies move at right angle to each other.

Feel the extremely difficult concepts that you never seemed to understand too easily with the formulas curated for various concepts on Physicscalc.Com