Simple Pendulum Calculator
With the help of our free Simple Pendulum Calculator, you can get the time period or length of the pendulum in a blink of an eye. All you need is to do is give acceleration due to gravity and pendulum length in the below input fields and press the calculate button to make your calculations easier and faster.
Simple Pendulum Calculator: Finding the simple pendulum time period and frequency is simple with this Simple Pendulum Calculator tool. From this page, you can learn the steps to calculate the period, frequency of a pendulum and formulas. Go through the further sections to get familiar with the simple pendulum topic. Know more about the concept and solved examples in the following sections.
How to Calculate Simple Pendulum Period?
The simple instructions to calculate the simple pendulum time period and frequency are mentioned here.
- Get the acceleration due to gravity and length of the pendulum.
- Divide the length by acceleration due to gravity and apply square root to the result.
- Multiply the value obtained in above step by 2π is called the time period.
- The reciprocal of time period is the frequency of the pendulum.
What is Simple Pendulum?
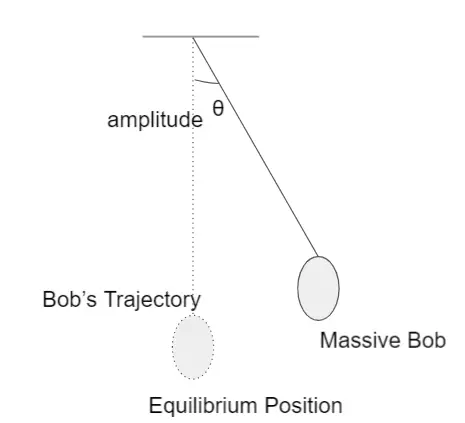
A simple pendulum is defined as the mass that is suspended from a rod. A straight vertical line passing through the fixed position is the pendulum mean position.
The important points about the simple pendulum are listed here:
- The distance between the centre of mass to the point of suspension is called the length of the pendulum and it is denoted by L.
- The oscillatory motion of a simple pendulum is the to and fro motion of the pendulum periodically and the mid-point of the oscillation is the equilibrium.
- The time taken by the pendulum to complete one full oscillation is called the time period of the simple pendulum and it is denoted by T.
- The amplitude of a simple pendulum is the distance travelled by the pendulum from one side to the equilibrium position.
The formulas to compute the simple pendulum period and frequency are provided below:
T = 2π × √(L/g)
f = 1/T
Where,
T is the time period of the pendulum
f is the frequency of the pendulum
L is the length of the pendulum
g is the acceleration due to gravity.
Example
Question: If the length of the simple pendulum is 15 m, find the time period and frequency of pendulum?
Solution:
Given that
The length of simple pendulum L = 20 cm
Acceleration due to gravity g = 9.8 m/s
Time Period T = 2π × √(L/g)
T = 2π × √(15/9.8)
= 2π × √(15/9.8)
= 2π × √(1.5306)
= 2 x 3.14 x 1.23
= 7.769
frequency f = 1/T
f = 1/7.769
= 0.128
Therefore, the ime period and frequency of simple pendulum is 7.769 seconds and 0.128 oscillations per second.
Not only Simple Pendulum Calculator you can also get other physics calculators on our site Physicscalc.Com to solve your physics problems at a faster pace while doing assignments or homeworks.
Frequently Asked Questions on Simple Pendulum Calculator
1. What are the applications of the simple pendulum?
The real-time applications of a simple pendulum are clock, wrecking ball, ballistic pendulum, bowling ball, Foucault's pendulum, and metronome.
2. What are the three laws of the simple pendulum?
The following are the three laws of a pendulum.
- The period of oscillation of a pendulum having constant length is independent of its amplitude.
- The period of oscillation of a simple pendulum of constant length is independent of the pendulum shape, size and material of the bob.
- The time period of a pendulum is directly proportional to the square root of the length of the simple pendulum.
3. What is the formula to compute the length of the pendulum?
The formula to find the length of the simple pendulum is L = (T²g)/(4π²).
4. What is the oscillation in the pendulum?
Oscillation means a pendulum that moves from one extreme position to the other extreme position and returns to the starting position. The time to complete one oscillation is called the time period.