Vector Formulas

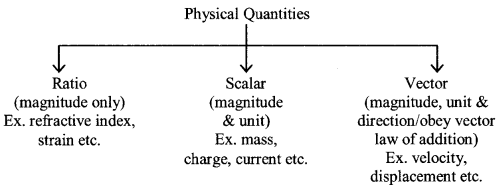
Types Of Vector

• Zero vector, \(|\overrightarrow{\mathrm{A}}|\) = 0
• Proper vector, \(|\overrightarrow{\mathrm{A}}|\) Γëá 0
• Like vector ΓåÆ same direction and same sense
• Unlike vector ΓåÆ not same sense
• Equal vector ΓåÆ same magnitude, same direction and same sense
• Negative vector ΓåÆ same magnitude but opposite direction
• Unit vector (vector of unit magnitude) ΓåÆ \(\hat{\mathrm{A}}=\frac{\overrightarrow{\mathrm{A}}}{|\overrightarrow{\mathrm{A}}|}\)

Note – \(\hat{i}, \hat{j} \text { and } \hat{k}\) are the unit vectors in the direction of x, y and z axis.

Representation Of Vector

\(\overrightarrow{\mathrm{A}}\) = \(A_{x} \hat{i}+A_{y} \hat{j}+A_{z} \hat{k}\)
Magnitude = \(|\vec{A}|=\sqrt{A_{x}^{2}+A_{y}^{2}+A_{z}^{2}}\)

Graphical method:

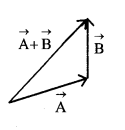
If \(\overrightarrow{\mathrm{A}}=\mathrm{A}_{\mathrm{x}} \hat{\mathrm{i}}+\mathrm{A}_{\mathrm{y}} \hat{\mathrm{j}}+\mathrm{A}_{\mathrm{z}} \hat{\mathrm{k}}\)
and \(\overrightarrow{\mathrm{B}}=\mathrm{B}_{\mathrm{x}} \hat{\mathrm{i}}+\mathrm{B}_{\mathrm{y}} \hat{\mathrm{j}}+\mathrm{B}_{\mathrm{z}} \hat{\mathrm{k}}\)
then \(\overrightarrow{\mathrm{R}}=\overrightarrow{\mathrm{A}}+\overrightarrow{\mathrm{B}}\) = (A_x + B_x)\(\hat{\mathrm{i}}\) + (A_y + B_y)\(\hat{\mathrm{j}}\) + (A_z + B_z)\(\hat{\mathrm{k}}\)
R = \(|\vec{A}+\vec{B}|=\sqrt{\left(A_{x}+B_{x}\right)^{2}+\left(A_{y}+B_{y}\right)^{2}+\left(A_{z}+B_{z}\right)^{2}}\)
Note: More than two vector also can be added similar to the above method.

Mathematical method:

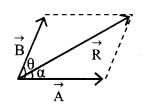
If \(|\overrightarrow{\mathrm{A}}|\) = A and \(|\overrightarrow{\mathrm{B}}|\) = B
θ → angle between \(\overrightarrow{\mathrm{A}} \text { and } \overrightarrow{\mathrm{B}}\)
then R = \(\sqrt{A^{2}+B^{2}+2 A B \cos \theta}\)
tan ╬▒ = \(\frac{B \sin \theta}{A+B \cos \theta}\)

Scalar Product

• \(\overrightarrow{\mathrm{A}} \cdot \overrightarrow{\mathrm{B}}\) = AB cos ╬╕
• \(\overrightarrow{\mathrm{A}} \cdot \overrightarrow{\mathrm{B}}=\overrightarrow{\mathrm{B}} \cdot \overrightarrow{\mathrm{A}}\)
• \(\overrightarrow{\mathrm{A}} \cdot \overrightarrow{\mathrm{B}}\) = A_xB_x + A_yB_y + A_zB_z
• \(\overrightarrow{\mathrm{A}} \cdot \overrightarrow{\mathrm{A}}\) = A²
• \(\hat{i} \cdot \hat{i}=\hat{j} \cdot \hat{j}=\hat{k} \cdot \hat{k}=1\)
• \(\overrightarrow{\mathrm{A}} \cdot \overrightarrow{\mathrm{B}}=0\)
• \(\hat{i} \cdot \hat{i}=\hat{j} \cdot \hat{j}=\hat{k} \cdot \hat{i}=0\)

⊥ condition

Vector Product

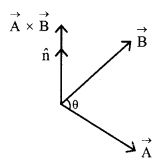
• \(\vec{A} \times \vec{B}\) = AB sin ╬╕ \(\hat{\mathrm{n}}\)
• \(\overrightarrow{\mathrm{A}} \times \overrightarrow{\mathrm{B}}=-\overrightarrow{\mathrm{B}} \times \overrightarrow{\mathrm{A}}\)
• \(\overrightarrow{\mathrm{A}} \times \overrightarrow{\mathrm{B}}\) = 0, || condition
• \(\overrightarrow{\mathrm{A}} \times \overrightarrow{\mathrm{B}}=\left|\begin{array}{ccc}
  \hat{\mathrm{i}} & \hat{\mathrm{j}} & \hat{\mathrm{k}} \\
  \mathrm{A}_{\mathrm{x}} & \mathrm{A}_{\mathrm{y}} & \mathrm{A}_{\mathrm{z}} \\
  \mathrm{B}_{\mathrm{x}} & \mathrm{B}_{\mathrm{y}} & \mathrm{B}_{\mathrm{z}}
  \end{array}\right|\)
  = \(\hat{\mathrm{i}}\) (A_yB_z – A_zB_y) + \(\hat{\mathrm{j}}\) (A_zB_x – A_xB_z)+ \(\hat{\mathrm{k}}\) (A_xB_y – A_yB_y)
  

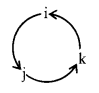
• \(\hat{\mathrm{i}} \times \hat{\mathrm{i}}=\hat{\mathrm{j}} \times \hat{\mathrm{j}}=\hat{\mathrm{k}} \times \hat{\mathrm{k}}=0\)
• \(\hat{\mathrm{i}} \times \hat{\mathrm{j}}=\hat{\mathrm{k}}, \hat{\mathrm{j}} \times \hat{\mathrm{k}}=\hat{\mathrm{i}}, \hat{\mathrm{k}} \times \hat{\mathrm{i}}=\hat{\mathrm{j}}\)

Direction Cosines

Direction cosines:

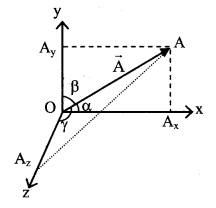
cos ╬▒, cos ╬▓ and cos ╬│ are called direction cosines in x direction, y direction and z direction respectively
cos a = \(\frac{A_{x}}{A}\), cos ╬▓ = \(\frac{A_{y}}{A}\) and cos ╬│ = \(\frac{A_{z}}{A}\) A A ‘A

• cos² ╬▒ + cos² ╬▓ + cos² ╬│ = 1
• sin² ╬▒ + sin² ╬▓ + sin² ╬│ = 2

Application Of Vector In Physics

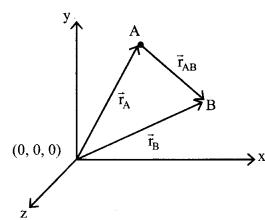
(1) Position vector (\(\vec{r}\)) of point w.r.t. origin (x, y, z): – \(\vec{r}\) = \(x \hat{i}+y \hat{j}+z \hat{k}\)

(2) Displacement vector \(\left(\overrightarrow{\mathrm{r}}_{\mathrm{AB}}\right)\) from point A (x₁, y₁, z₁) to B (x₂, y₂, z₂) \(\overrightarrow{\mathrm{r}}_{\mathrm{AB}}=\overrightarrow{\mathrm{r}}_{\mathrm{B}}-\overrightarrow{\mathrm{r}}_{\mathrm{A}}\) = (x₂ – x₁)\(\hat{\mathrm{i}}\) + (y₂ – y₁ )\(\hat{\mathrm{j}}\) + (z₂ – z₁)\(\hat{\mathrm{k}}\)

(3) Relative velocity: \(\overrightarrow{\mathrm{V}}_{\mathrm{AB}}=\overrightarrow{\mathrm{V}}_{\mathrm{B}}-\overrightarrow{\mathrm{V}}_{\mathrm{A}}\)
Example of dot product
Work: W = \(\overrightarrow{\mathrm{F}} \cdot \overrightarrow{\mathrm{r}}\), Power P = \(\overrightarrow{\mathrm{F}} \cdot \overrightarrow{\mathrm{v}}\)
Φ_E = \(\overrightarrow{\mathrm{E}} \cdot \overrightarrow{\mathrm{A}}\), Φ_B = \(\overrightarrow{\mathrm{B}} \cdot \overrightarrow{\mathrm{A}}\)
Example of cross product
\(\overrightarrow{\mathrm{v}}=\vec{\omega} \times \overrightarrow{\mathrm{v}}, \overrightarrow{\mathrm{L}}=\overrightarrow{\mathrm{r}} \times \overrightarrow{\mathrm{P}}, \quad \vec{\tau}=\overrightarrow{\mathrm{r}} \times \overrightarrow{\mathrm{F}},\) \(\overrightarrow{\mathrm{F}}=\mathrm{q}(\overrightarrow{\mathrm{v}} \times \overrightarrow{\mathrm{B}})\)

• \(\overrightarrow{\mathrm{v}}=\mathrm{d} \overrightarrow{\mathrm{r}} / \mathrm{dt}, \overrightarrow{\mathrm{a}}=\mathrm{d} \overrightarrow{\mathrm{v}} / \mathrm{dt}\)
• Lorentz force: \(\overrightarrow{\mathrm{F}}=\mathrm{q}(\overrightarrow{\mathrm{E}}+\overrightarrow{\mathrm{v}} \times \overrightarrow{\mathrm{B}})\)
• Area of a triangle: \(\frac{1}{2}|\overrightarrow{\mathrm{A}} \times \overrightarrow{\mathrm{B}}|\)
• Area of a parallelogram: \(|\overrightarrow{\mathrm{A}} \times \overrightarrow{\mathrm{B}}|\)
• Volume of a parallelepiped: \(\overrightarrow{\mathrm{A}} \cdot(\overrightarrow{\mathrm{B}} \times \overrightarrow{\mathrm{C}})\)
• Gradient operator: Γêç = \(\hat{\mathrm{i}} \frac{\partial}{\partial \mathrm{x}}+\hat{\mathrm{j}} \frac{\partial}{\partial \mathrm{y}}+\hat{\mathrm{k}} \frac{\partial}{\partial \mathrm{z}}\) Ex. \(\overrightarrow{\mathrm{E}}\) = -Γêç╬ª

Grab the opportunity to get all the Physics Concepts Formulas along with Vectors Formulae at one go from Physicscalc.Com and clarify all your queries.